John Bardeen and the Theory of Superconductivity
by Lillian Hoddeson (1)
Introduction
Every theory of superconductivity can be disproved! This tongue-in-cheek theorem struck a chord when Felix Bloch announced it in the early 1930s. Virtually every major physicist then working on theory — including, besides Bloch, Niels Bohr, Wolfgang Pauli, Werner Heisenberg, Lev Landau, Leon Brillouin, W. Elsasser, Yakov Frenkel, and Ralph Kronig — had tried and failed to explain the mysterious phenomenon in which below a few degrees Kelvin certain metals and alloys lose all their electrical resistance.(2) The frequency with which Bloch's theorem was quoted suggests the frustration of the many physicists who were struggling to explain superconductivity.
Neither the tools nor the evidence were yet adequate for solving the problem. These would gradually be created during the 1940s and 50s, but bringing them to bear on superconductivity and solving the long-standing riddle required a special set of talents and abilities: a deep understanding of quantum mechanics and solid state physics, confidence in the solubility of the problem, intuition about the phenomenon, a practical approach to problem-solving, patience, teamwork, and above all refusal to give up in the face of repeated failures. When John Bardeen took on the problem of superconductivity in the late 1930s, he held it like a bulldog holds a piece of meat, until he, his student J. Robert Schrieffer, and his postdoc Leon Cooper solved it in 1957.
Princeton and Harvard
Bardeen probably first encountered the problem of explaining superconductivity between 1933 and 1935, when he was a graduate student at Princeton. He was entering the new field of the quantum theory of solids and avidly reading its pioneering papers. In their comprehensive review published in the 1933 Handbuch der Physik, Hans Bethe and Arnold Sommerfeld identified superconductivity as the only solid state problem that still resisted treatment by the quantum theory.(3) While we have no evidence Bardeen even attempted to attack the problem in that period, he likely entertained the thought, for he was amply endowed with competitive spirit.
Arriving at Princeton in the fall of 1933, in the depths of the Great Depression, Bardeen boldy turned his back on the secure engineering post he had held for the last three years at Gulf Research Laboratory in Pittsburgh. He enrolled in Princeton's graduate program in mathematics. Abandoning his initial idea of working with Einstein, who also arrived in Princeton that fall, Bardeen became the second graduate student of the young, but already quite eminent, mathematical physicist, Eugene Wigner.
Just then, Wigner was excited about employing quantum mechanics to explain the multitude of behaviors and properties of real materials. He was working with his first graduate student, Frederick Seitz, on developing a simple approximation method for calculating the energy bands of sodium, the first real (i.e., nonideal) material to which the quantum theory of metals was applied. Wigner was bothered by the fact that his work with Seitz failed to account for the interactions between electrons. He recognized that his own attempts to add an electron interaction term in a study of the cohesive energy of metals was only the beginning of the development of a "many-body" theory, in which the interactions between electrons, as well as between the electrons and lattice are properly dealt with.(4)
Wigner posed the fundamental question to Bardeen: How do the electrons inside metals interact? The problem so enticed the student that he never let go of it throughout his physics career of almost 60 years. He returned to it, for example, in his doctoral thesis, in which he calculated a metal's "work function" (the energy needed to remove an electron from the metal),(5) in his study of semiconductor surface states in 1946, a major step in the invention of the transistor;(6) and in the numerous many-body problems he addressed from the 1950s on, including charge density waves and superconductivity.
During Bardeen's period as a Harvard Junior Fellow from 1935 to 1938, he often found himself frustrated by problems that required a many-body theory. For instance, he was unable to explain the experimental finding that the "Fermi surface" (the surface of the Fermi-Dirac distribution in wave vector space) is sharp, despite exchange and correlation effects, as suggested by the recent experiments at MIT of Henry O'Bryan and Herbert Skinner.(7) While Bardeen recognized that correlation effects had to be taken into account to avoid having an infinite velocity at the Fermi surface, he did not know how to correctly include them in the calculation.(8) The process of working on many-body problems that could not yet be solved within the existing theoretical framework helped Bardeen prepare for the major challenge of his career.
While he did not yet take a real stab at explaining superconductivity while he was at Harvard, Bardeen later claimed that he became interested in the problem there in the course of studying the new phenomenological theory published in 1935 by the London brothers, Fritz and Heinz, who had resettled at Oxford after fleeing Hitler's Germany.(9) Bardeen was powerfully drawn to this theory, particularly to its idea that superconductivity exists as a macroscopic quantum state — "the superconductor become characterized as a single large diagmagetic atom."(10) Bardeen believed this intuitively: although determined by an ordering of electrons extending over substantial distances (10-4 cm), the state of superconductivity required a quantum-mechanical description. To fully establish this intuition would take Bardeen approximately two decades.
Minnesota
Bardeen began his work on superconductivity at the University of Minnesota, where he held his first academic post from 1938 to 1941. To get a "feel" for the phenomenon, he read David Shoenberg's new book reviewing the experimental situation.(11) Experiments established that the transition to superconductivity is reversible and can therefore be described using thermodynamics. Most shocking was Walther Meissner's and Robert Ochsenfeld's experimental finding in 1933 that superconductors expel magnetic fields. Ever since Heike Kamerlingh Onnes's discovery of superconductivity in 1911, zero resistance had been considered the essential feature of superconductivity. Now it appeared that diamagnetism might be more basic. The vanishing of the resistivity followed mathematically from the London theory, which had been modeled phenomenologically to account for the expulsion of magnetic field. Bardeen felt it would be possible to derive the London theory from first principles.
He tried viewing the experiment of Meissner and Ochsenfeld from the point of view of the electrons in the lattice, asking whether the Meissner effect could mean that electron orbits are much larger in superconductors than anyone had realized. Seeking to explain in a quantum-mechanical framework how gaps appear in the electronic structure, as stressed by the Londons, he drew on the Pauli exclusion principle and guessed that because the energy scale of superconductivity is low (about 10-4 eV) the only electrons likely to be involved are those at the edge of the Fermi surface. (Electrons further in would not have states to receive them.) Like an engineer testing his apparatus, he tapped his theoretical model and explored introducing a small periodic distortion of the crystal lattice.
In one of his more important works at Harvard, a first-principles calculation of the electron-phonon interaction in metals, Bardeen had assumed (unlike earlier calculations) that the unscreened potential moves along with the ion. Applying ideas he developed there to superconductivity, Bardeen tried to show that a periodic disturbance introduced into a superconductor causes the electrons to gain an amount of energy which more than compensates for losses due to ionic displacement. From the disparity he hoped to explain how the gaps form.(12) Unfortunately the numbers were off by more than a factor of ten; he did not commit his calculation to print (other than as an abstract). Bardeen could not help but recognize that his work was only a beginning.
He would have to wait almost a decade to continue the study, for in March 1941 he was suddenly called to Washington D.C. to work during World War II at the Naval Ordnance Laboratory on magnetic mines. But, he later confessed, "The concept of somehow getting a small energy gap at the Fermi surface remained in the back of my mind."(13)
Bell Labs
Bardeen moved in October 1945 to Bell Telephone Laboratories, where he joined a new semiconductor group directed by William Shockley. Bardeen initially enjoyed working in this group, until the atmospher changed in late December 1947, after he and Walter Brattain invented the first transistor, a point-contact device. Chagrined not to have been directly involved in the discovery, Shockley now began feverishly to pursue the original transistor's sequel, the junction transistor, excluding Bardeen and Brattain and generally ruining the quality of their research life.(14)For two years, Bardeen tried to work in this frustrating environment. By early 1950, he knew he was wasting his time. "Bardeen was fed up with Bell Labs — with a particular person at Bell Labs," Brattain reflected.(15) Bardeen's efforts to separate himself from the pain of working under Shockley brought him to the most important work of his life.
Bardeen pulled out his old notes on superconductivity. Reviewing the experimental progress made since he last worked on the problem, he noticed that much new evidence was supporting the London theory.(16) But what riveted him to the problem was a phone call he received on May 15, 1950 from Bernard Serin. The Rutgers experimentalist wanted to speak with Bardeen about his new findings studying mercury isotopes, available as a consequence of the wartime atomic bomb program. Examining isotopes made at Oak Ridge having mass numbers between 198 and 202, Serin and his students had found an "isotope effect," the lighter the mass, the higher the temperature at which the materials turn superconducting. Emanuel Maxwell at the National Bureau of Standards found the same effect independently studying isotopes made at Los Alamos.
Bardeen instantly understood the new clue these results offered, noting to himself on May 16th, "electron-lattice interactions are important in determining superconductivity." He spent the next several days trying lattice fluctuations in place of the periodic lattice distortion in his Minnesota theory. The effort failed, but he was sure he was on the right path. To secure priority, he dashed off a letter to the Physical Review outlining the idea.(17)
As it happened, Bardeen was not the only theorist to connect superconductivity with the electron-lattice interaction. Earlier in 1950, before Maxwell and Serin found the isotope effect experimentally, Herbert Fröhlich had set forth a theory predicting it. When Fröhlich learned of the expermental results a day or two after they appeared in the Physical Review, he sent a letter to the Proceedings of the Royal Society to claim priority for his theory.(18) The competition was on.
Neither Fröhlich nor Bardeen could calculate all the relevant quantities, such as the superconducting wave function, the energy of the superconducting state, or the effective mass of the electrons. Their mathematical formalism was too limited. While both theories could explain the isotope effect, they could not explain superconductivity because they focused on individual electron energies rather than the energy that arises from the interaction of many electrons. The basic problem on which both got stuck was to find an interaction that made the total energy of the superconducting state lower than that of the normal state. The energy from the electron-phonon interaction had to dominate that arising from the ordinary Coulomb repulsion of electrons. More than a year later, Bardeen confessed to Rudolf Peierls that all the methods he had tried could not treat this problem. Even so, he wrote,"I believe that the explanation of the superconducting properties is to be found along the lines suggested by F. London." The hint that bolstered Bardeen's confidence was, "The wave functions for the electrons are not altered very much by a magnetic field."(19) This "rigidity" of the wave functions, assumed by the Londons, offered a basis for the long-range ordering.
Meanwhile Bardeen increasingly felt like an outcast at Bell Labs. He longed for greater contact with colleagues, students, and especially experimentalists, not to mention institutional support for his research on superconductivity. Shockley was a continuing source of irritation. During a fall conference in the Pocono Mountains, Bardeen sat down with his old Princeton friend and colleague, Frederick Seitz, for a heart-to-heart talk. He told Seitz about his problems with Shockley and about his exciting work on superconductivity. "I'm really planning to leave the Bell Labs, can you advise me of any jobs?"(20)
Seitz was the perfect confidant. Not only had he known Shockley for many years, but he was just then building a solid state group at the University of Illinois. Seitz spoke with administrators and soon Illinois extended an offer to Bardeen, who responded, "well Illinois would be perfect, it's the kind of place I'd like to be at."(21)
Illinois
After the move to Illinois, Bardeen prepared to finally crack the riddle of superconductivity. Starting over, he approached the problem in the way Wigner taught him, separating it into smaller parts, examining all manageable pieces, later trying to reassemble the parts to get a handle on the larger issue.(22) He soon encountered the old hurdle of the many-body interactions. He was aware that in using the standard (Hartree) approximation, he might be eliminating the most critical aspect.
Bardeen also made another move of a kind that had served him well in previous projects, including his work on the transistor. He engaged collaborators who had knowledge, talents, or experiences that he judged possibly relevant and that he himself lacked. He thought David Bohm's new many-body formalism for treating the electron plasma might be useful in modeling the electron-electron interactions. Bohm's interest in electron plasmas grew out of his wartime work on electromagnetic separation of isotopes.(23) Bardeen was particularly interested in the way Bohm and his student David Pines had mathematically separated the troublesome long-range Coulomb interactions from the single-particle excitations, which interact short-range. Offering Pines a postdoctoral position at Illinois, Bardeen hoped to extend his own repertoire with Pines' experience.
When Pines arrived in July 1952, Bardeen asked him to look at a problem Fröhlich had recently studied, the motion of an electron in a polar crystal. Simpler than superconductivity, this "polaron" problem, had a number of the same features. One could study in a less complex system how the electrons are strongly coupled to the lattice vibrations (phonons). Working with Tsung-Dao Lee, a young theorist then spending the summer in Urbana as Bardeen's postdoc, Pines realized that a method Lee had recently used in his field theory studies (the "intermediate coupling method") could be adapted for the polaron problem.(24) Also bringing in Francis Low, then on the Illinois faculty, Lee, Low, and Pines arrived at a formulation that would be useful in the development of the BCS theory.
Then Bardeen worked with Pines to adapt the Bohm-Pines theory to treat the combined influence of all the electron interactions in a metal. In a calculation comparing the size of the attractive phonon-induced interaction with that of the repulsive Coulomb interaction, they found that for cases where the energy transfer is small, the attractive interaction is stronger.(25) Bardeen immediately recognized the importance of this finding: for pairs of electrons near to the Fermi surface, the net electron-electron interaction is attractive!
In the same period, Bardeen also undertook an extensive literature study of superconductivity while writing a review article on the theory for the 1956 Handbuch der Physik. In the review he argued for London's notion of superconductivity as an "ordered phase in which quantum effects extend over large distances in space" and ventured that superconductors are "probably characterized by some sort of order parameter which goes to zero at the transition." But, he admitted, "we do not have any understanding at all of what the order parameter represents in physical terms."(26) He emphasized the diamagnetic origin of supercurrents, and discussed the second-order phase transition between the normal and superconducting state. Following London, he stressed the role of the energy gap caused by the rigidity of the wave function with respect to magnetic perturbation. While he could not yet derive the gap, by assuming it, he could show how to develop both the electrodynamic properties of superconductors and a generalization of the London equations similar to the non-local formulation of superconductor electrodynamics recently put forth by Pippard.
Another focus was the machinery for computing both the electron-electron and electron-phonon interactions. He stressed the importance of considering the electrons as electrically "screened," and he commented on the promise offered by recently developed field theoretical techniques, such as Sin-itiro Tomonaga's strong-coupling approach and the Bohm-Pines theory. He concluded: "A framework for an adequate theory of superconductivity exists, but the problem is an exceedingly difficult one. Some radically new ideas are required."(27)
Painfully aware of Fröhlich's advantage in field theory, Bardeen telephoned Chen Ning Yang at the Princeton Institute for Advanced Study during the Spring of 1955 and asked whether he could send to Urbana someone "versed in field theory who might be willing to work on superconductivity."(28) Yang recommended Leon Cooper, who had recently taken his Ph.D. After arriving in September, the young theorist offered a series of seminars on field theory. The third member of the team, J. Robert Schrieffer, was a Bardeen graduate student who selected superconductivity for his thesis after proofreading Bardeen's Handbuch article because superconductivity "looked like the most exciting thing."(29)
Bardeen was unquestionably the leader who set the problems, motivated the members, organized the approach, and planted theoretical seeds by making appropriate assignments. He asked Schrieffer to look into the "t-matrix methods" that Keith Brueckner recently developed in studying nuclei. He asked Cooper to examine the Bohm-Pines theory, as well as his 1954 work with Pines on the electron-electron interaction. Bardeen continued to look out for other useful leads, while nurturing the team's work in frequent discussions.
The collaboration was family-style. Bardeen and Cooper shared an office. And when Schrieffer came to speak with either, both would "wheel around their chairs" and join in. Schrieffer claims that he and Cooper absorbed Bardeen's taste in physics, his experiment-based methodology, his habit of breaking down problems, and his simple style of using as little theoretical machinery as possible, "the smallest weapon in your arsenal to kill a monster."(30)
As the team grappled with the difficult many-body problem, Bardeen held to his belief that the key to solution was in the London theory, which Fritz London had recently reformulated in a book that explained better how the rigidity of the wave function and the long-range ordering brought about "a quantum structure on a macroscopic scale ... a kind of solidification or condensation of the average momentum distribution,"(31) Another guiding idea was that there is only one stable current distribution, and in thermal equilibrium there is no persistent current in an isolated superconductor, unless the system is in the presence of a magnetic field. Bardeen further stressed that these currents "differ for every variation of the strength or direction of the applied field." Schrieffer recalls Bardeen pressing them to clarify the notion of long-range order using a "phase coherence" parameter of the size (the order of a micron) of typical correlations between the particles.
Bardeen also helped the team strke out into the unknown by offering a principle that formed a bridge between the known theory of the normal state and the unknown theory of superconductivity. The principle stated that the superconducting energy states should correspond one-to-one with the normal states. Thus it should be possible to express the wave function of the superconducting state as a linear sum of the normal state functions as defined in quantum field theory. That way of thinking helped them concretize their meditations and concentrate on the small energy difference between the normal and superconducting states.(32)
Cooper had a breakthough in September 1956. Examining the simple case of only two electrons just outside the Fermi surface, and making certain other assumptions, he showed that if the net force between them is attractive, when their energies lie within a certain range of one another the two electrons form a bound state below the continuum states that is separated from them by an energy gap.(33) But the group got stuck trying to go from a single "Cooper pair" to a many-electron theory. A major difficulty was coping with the fact that many pairs would overlap. Schrieffer later portrayed the problem using an analogy with couples dancing the Frug on a crowded floor. Even though partners dance apart for considerable periods, and even though other dancers come between, each pair remains a couple. The problem was to represent that situation mathematically.(34)
They worried about their approximations. The energy change in the transition from normal to superconducting (about 10-8 eV per electron) was much smaller than the accuracy with which they could calculate the energy of either state. In working only with the part of the system responsible for pairing, they knew they might be ignoring another part important enough to invalidate the whole analysis.
They still were stuck in November when the exciting news broke that Bardeen, Brattain, and Shockley had won the 1956 physics Nobel Prize for the invention of the transistor. This was a most confusing time for Schrieffer. Now a fourth-year student, he had recently been offered an attractive NSF fellowship that he wanted to accept for study in Europe. But a condition was that he be done with his doctorate. Schrieffer was pleased about Bardeen's prize, but he had his own future to consider. He met with Bardeen shortly before the latter's trip to Sweden and asked, since the group was at an impasse, whether it might make sense for him to switch his thesis problem.(35)
Bardeen did not want to slow his student's career, but he truly read the situation differently. Having worked on superconductivity for almost two decades, he could sense what Schrieffer could not: that they were very close to breakthough, so close that he could not let him give up. "Give it another month, or a month and a half," he muttered. "Wait 'til I get back and keep working. Maybe something'll happen and we can discuss it a little later."(36)
The timing of the Nobel was in fact poor for Bardeen too. Richard Feynman had spoken on superfluidity and superconductivity that September. Bardeen was well aware of Feynman's advantage in field theory. And on some deep level, he felt that from a physics point of view the transistor, although important technologically, was only a gadget.(37)
Bardeen went right back to work after Stockholm. His daughter Betsy, then 13, recalled that during Christmas her father was in another world.(38) Yet the problem did not break in December, nor through most of January. But in the last days of January the turn came. Schrieffer and Cooper were attending meetings on the many-body problem on the East Coast, one in Hoboken and another in New York City. As Schrieffer was commuting between the meetings, and also to Summit, New Jersey, where he was staying with a friend, something clicked.
The process, as Schrieffer remembered, was a sort of intellectual tinkering. Having listened to talks on the nuclear interaction (between pi-mesons, protons and neutrons) and thinking constantly about superconductivity, he ventured to guess a possible form of the wave function for the superconducting ground state, one that took the Cooper pairs into account. Then he tuned up the expression using a variational approach like the one Tomonaga had used in the pion-nucleon problem. Knowing that a conventional (Hartree) product, where the state k is either occupied or unoccupied, does not lead to an energy lowering, he made sure his wave function didn't require any given state to be definitely occupied or unoccupied. "I wanted to have some flexibility, so the electrons could scatter around and lower their energy."
He called on Bardeen's bridging principle, "to form the wave function as a coherent super-position of normal state-like configurations." Tinkering on, he realized, "so many pairs, they're overlapping — some sort of a statistical approach is appropriate." Following Tomonaga, he tried forming a product, thinking, "Well at least that allows the pairs to hop from state to state, and that seems like a reasonable guess." He noticed that what he had constructed didn't conserve the number of electrons, and when he tried to fix that problem, "I decided what I should do is multiply that wave function by a term involving E to the minus the number of particles," in effect employing what in statistical mechanics is known as the grand canonical ensemble.(39)
Then "it all sort of crystallized" while he was on the subway. "I scribbled down the wave function and calculated the begining of that expectation value, and I realized that the algebra was very simple." He worked more on the expression that night at his friend's house, and in the morning did a variational calculation to determine the gap equation. "I solved the gap equation for the cutoff potential. It was just a few hours work." Expanding the product, he found he had written down a product of mathematical operators on the vacuum that expressed the creation of electrons. In his sum of a series of terms, each one corresponded to a different total numbers of pairs. He was completely astonished to find that his expression "was really ordered in momentum space" and that the ground state energy "was exponentially lower in energy," as required for the state to be stable.(40)
Schrieffer could hardly wait to tell Bardeen and Cooper. By chance he and Cooper flew into Champaign at the same time, and he could not resist showing the expression to Cooper right there in the airport. "Great, looks terrific," Cooper said. "Let's go and talk to John in the morning."(41) And when Bardeen saw the wave function, he calmly drawled that "he thought that there was something really there." Then, after "we chatted around about that for a few hours," Bardeen set out to try to use the wave function to compute the energy gap. Schrieffer remembered that Bardeen was very confident and that it took him only a few days. The magnitude with the gap parameter in the ground state energy!(42)
The most exciting moment occurred several days later, when Bardeen calculated the condensation energy in terms of both the energy gap and the critical field, obtaining a relationship between these experimentally determined quantities. At first Bardeen had trouble converting units. He "was very upset that he couldn't get the numbers to work out." But eventually they did work and turned out "something like 9 compared to 11 in the appropriate units. And we were really overjoyed, and sort of hit the roof. Things looked like pay dirt."(43) All the pieces were fitting together.
The three began to race. Bardeen divided the tasks asing Schrieffer to work on thermodynamic properties, Cooper to explore the Meissner effect and other electrodynamic properties, while he took on the transport and non-equilibrium properties. Bardeen's colleagues knew that something was up when they asked him a question and were told, apologetically, that he was too busy to think about anything else just then.(44)
Two weeks after Schrieffer's breakthough, they were ready to publish. But Bardeen had not succeeded in deriving the second-order phase transition. He finally decided not to let this hold up their publication any longer. When they sent their historic letter on BCS to the Physical Review on February 15th, Bardeen requested immediate publication: "I know that you object to letters, but we feel that this work represents a major breakthrough in the theory of superconductivity and this warrants special handling."(45) And shortly after sending off the letter, Bardeen succeeded in computing the second-order phase transition.
The letter explained how superconductivity arises from the coupling between electrons and phonons, an interaction in whose presence the system forms a coherent superconducting ground state in which individual particle states are occupied in pairs, "such that if one of the pair is occupied, the other is also."(46) The letter summarized the advantages of the theory:
- It leads to an energy-gap model of the sort that may be expected to account for the electromagnetic properties.
- It gives the isotope effect.
- An order parameter, which might be taken as the fraction of electrons above the Fermi surface in virtual pair states, comes in a natural way.
- An exponential factor in the energy may account for the fact
that kTc is very much smaller than
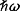 .
. - The theory is simple enough so that it should be possible to make calculations of thermal, transport, and electromagnetic properties of the superconducting state.
Bardeen announced the breakthough to his Illinois colleagues in a characteristic way. Bumping into Charles Slichter in the hall, he momentarily struggled for words, and then offered, "Well, I think we've figured out superconductivity." Slichter remembers that instant as "the most exciting moment of science that I've ever experienced."(47)
Slichter and his student Charles Hebel were among the first to confirm the BCS theory experimentally. Measuring the rate at which nuclear spins relax in aluminum as a function of temperature, they found that as they lowered the temperature and the aluminum makes its transition to superconductivity the nuclear magnetic resonance rate increases, instead of decreasing, to more than twice its value in the normal state. Then as the temperature is further reduced, the rate begins to decrease again. While the effect was contrary to the predictions of the prevailing (two-fluid) model of superconductivity, BCS could explain it in terms of an increased density of states below the transition temperature. Soon many experiments at many institutions were confirming the theory.(48)
The team announced the theory at the annual solid state meeting of the American Physical Society in March, held that year in Philadelphia. Concerned that Schrieffer and Cooper receive their due credit, Bardeen decided not to attend and arranged for two post-deadline papers to be delivered by his younger teammates. Schrieffer got word too late to attend, so Cooper had to deliver both papers. One week later, their historic letter on the BCS theory appeared in the Physical Review.
Their full-length article, sent to the Physical Review four months later, showed in more detail how the theory explains: (1) the infinite conductivity discovered by Kamerlingh Onnes; (2) the diamagnetic effect found by Meissner and Ochsenfeld; (3) the second-order phase transition at the critical temperature; (4) the isotope effect; and (5) the energy gap. It also showed how the theory gives quantitative agreement for other experimentally determined quantities including the specific heat and penetration depth.(49)
Many theorists met the theory with criticisms or questions. One objection concerned the apparent lack of gauge invariance. When Philip Anderson, Pines, Schrieffer, and others dealt with this issue, their work had an important by-product, the idea of "broken symmetry." One of the original objectors to BCS, Yoichiro Nambu, then introduced the notion into particle physics, where it helped build the Standard Model of particles and fields.(50)
Bardeen worried that the Swedish Academy of Sciences would keep to its tradition of not awarding any individual two Nobel Prizes in the same field, thus preventing Schrieffer and Cooper from receiving an award they had earned. But to his relief and joy, the Academy broke with precedent and honored all three with the 1972 Nobel Prize for Physics. Bardeen has the distinction of being the first person to win two Nobel Prizes in the same field.
Acknowledgements
Quin Luttinger, my doctoral advisor, did not share my passion for history of physics. Although he was amused by some of the details, he was painfully aware of the human complexities behind the scene and doubted anyone could get them right. For a physics graduate student, he considered history a frivolity and a distraction. As my advisor, it must have been frustrating to recognize that my interest in physics was driven by a desire to understand its history. Quin did not hesitate to apply this insight. He knew I would work harder to grasp the physics of any assignment that included history. So in 1963 he devised an exercise to teach me something about superconductivity. He called for a paper discussing progress in the field during the last three decades. It was a great problem for me, one I thoroughly enjoyed. Unfortunately, when I recently stumbled across that old student work in a dusty carton, I was embarrased by its poor historical quality — which for Quin could only have confirmed his disapproval of history. I'd like to take this opportunity to resubmit a new draft, one more narrowly focused on the work of a single major actor and based on materials not available to me when I was a graduate student. While the account does not begin to represent the whole story, I think it is an improvement over what I did with that old assignment Quin was kind enough to design for me.
 |
| Lillian Hoddeson (with David Bartlett) inspecting the particle detector for his high-energy physics experiment at Columbia University's Nevis Laboratory in 1962, when she was a graduate student. |
I would like to thank Gordon Baym for a helpful critical reading of an earlier version of this article, and the Richard Lounsbery Foundation, the Alfred P. Sloan Foundation, the Dibner Fund, and the University of Illinois' Center for Advanced Study for their generous support of this historical work.
Notes
1. Reprinted from Journal of Statistical Physics vol. 103, nos. 3/4, 2001, pp. 625-640. This article draws in part on a manuscript by L. Hoddeson and V. Daitch, True Genius: the Life and Physics of John Bardeen, and Hoddeson, G. Baym, H. Schubert, S. Heims, "Chapter 8: Collective Phenomena," in Out of the Crystal Maze: A History of Solid State Physics, 1900-1960, edited by L. Hoddeson, E. Braun, J. Teichmann and S. Weart (New York: Oxford Univ. Press, 1992), pp. 489-616. An earlier version of this article was the basis of my article "John Bardeen and the BCS Theory of Superconductivity," in Materials Research Society Bulletin, Vol. 24, No. 1, pp. 50-55 (Jan. 1999). BACK
2. L. Hoddeson, G. Baym, and M. Eckert, "The Development of the Quantum Mechanical Electron Theory of Metals, 1926-1933," in Crystal Maze, op. cit., pp. 88-181, see esp. pp. 140-153.BACK
3. A. Sommerfeld and H. Bethe, "Elektronentheorie der Metalle," in H. Geiger and K. Sheel, eds., Handbuch der Physik, ser. 2, (Berlin: Springer, 1933), 24, pp. 333-622, quote on p. 555. BACK
4. See "Collective Phenomena," in Crystal Maze, p. 491. BACK
5. E. P. Wigner and J. Bardeen, "Theory of the Work Functions of Monovalent Metals," Physical Review 48 (1935), pp. 84- 87; J. Bardeen, "Theory of the Work Function, II The Surface Double Layer," Physical Review 49 (1936), pp. 653-663. J. Bardeen, "Reminiscences of Early Days in Solid State Physics," in The Beginnings of Solid State Physics, edited by Sir Nevill Mott (London: The Royal Society, 1980), pp. 77-83. BACK
6. See M. Riordan and L. Hoddeson, Crystal Fire: the Birth of the Information Age (New York: W. W. Norton, 1997). BACK
7. H. Jones, N.F. Mott, and H. W. B. Skinner, "A Theory of the Form of the X-ray Emission Bands and Metals, Physical Review, 45 (1934), 379-84. BACK
8. J. Bardeen, "Reminiscences," op. cit.BACK
9. Bardeen, "Reminiscences" op. cit.; F. London and H. London, "Supraleitung und Diamagnetismus," Physica 2 (1935), pp. 341-354; F. London, "Macroscopic Interpretation of Supraconductivity," Proc. Roy. Soc. A152 (1935), pp. 24-34. For a summary of research on superconductivity up to the Second World War, see P. F. Dahl, Superconductivity: Its Historical Roots and Development from Mercury to the Ceramic Oxides (New York: American Institute of Physics, 1992). For insight into the work of the London brothers, especially Fritz, see K. Gavroglu, Fritz London: a scientific biography (New York: Cambridge University Press, 1995). For further references see Crystal Maze, p. 588, n 21, pp. 141-53, and sec. IV. "Superconductivity, 1929-1933," in L. Hoddeson, G. Baym and M. Eckert, "The development of the quantum-mechanical theory of metals, 1928-1933," Rev. Mod. Phys. 59 (1987), 287-327. BACK
10. London and London, "Supraleitung und Diamagnetismus," p. 348. BACK
11. D. Shoenberg, Superconductivity (Cambridge: Cambridge University Press, 1938). In the mid-50s, Bardeen would recommend the 1952 edition of this book to his postdoc Leon Cooper. BACK
12. Bardeen, "Theory of Superconductivity," Abstract, Physical Review 59 (1941), 928. Bardeen would employ a similar approach in his work during the 1980s on charge density waves. BACK
13. J. Bardeen, "Reminiscences," op. cit.. BACK
14. This story is told in Crystal Fire, pp. 185-192. BACK
15. Brattain interview by C. Weiner, May 28, 1974, AIP, quoted in Crystal Fire, p. 190. BACK
16. The evidence came from work by a group of experimenters based in different labs including A. Brian Pippard, William Fairbank, Emanuel Maxwell, Paul Marcus, J. C. Daunt, Kurt Mendelssohn, B. Goodman, A. Brown, Mark Zemansky, Henry Boorse, W. Corak, Michael Tinkham, and R. E. Glover. BACK
17. Bardeen notes written between May 15 and May 18, 1950, Bardeen papers, Univ. of Illinois Archives; J. Bardeen, "Zero-Point Vibrations and Superconductivity," Physical Review, 79 (1950), pp. 167-68; Chapter 8, Out of the Crystal Maze, pp. 549-50. BACK
18. H. Fröhlich, "Isotope Effect in Superconductivity," (letter) Proceedings of the Physical Society (London) A63 (1950), p.778. BACK
19. Bardeen to Peierls, July 17, 1951, Peierls Papers, Oxford. BACK
20. F. Seitz interview by L. Hoddeson, V. Daitch, and I. Elichirigoity, April 22, 1993. BACK
21. Ibid. BACK
22. D. Pines interview with Lillian Hoddeson and Vicki Daitch, December 3, 1993, p. 8. BACK
23. D. Bohm interview by L. Hoddeson, May 8, 1981; see Crystal Maze p. 536. BACK
24. D. Pines interview by L. Hoddeson, August 13 and 16, 1981. BACK
25. J. Bardeen and D. Pines, "Electron-Phonon Interaction in Metals," Physical Review 99 (1955), pp. 1140-1150. BACK
26. J. Bardeen, "Theory of Superconductivity. Theoretical Part," in Handbuch der Physik (Berlin: Springer, 1956), Vol. 15, 274-369. BACK
27. Bardeen, "Theory of Superconductivity. Theoretical Part." BACK
28. J. Bardeen, "History of Superconductivity Research," in Impact of Basic Research on Technology, edited by B. Kursonoglu and A. Perlmutter (New York: Plenum, 1973), 15-57. BACK
29. Schrieffer interview with J. Warnow and R. M. Williams, Sept. 26, 1974, hereafter cited as SWW. BACK
30. SWW. BACK
31. F. London, Superfluids, vol. 1: Macroscopic theory of Superconductivity. (New York: Wiley, 1950), esp. 142-155. BACK
32. SWW. BACK
33. L. Cooper, "Bound Electron Pairs in a Degenerate Fermi Gas," Physical Review 104 (1956), 1189-1190. BACK
34. SSW. BACK
35. SWW. BACK
36. SWW. BACK
37. D. Lazarus interview with I. Elichirigoity, 24 February 1992. BACK
38. Betsy Bardeen Greytak, Bardeen family interview with L. Hoddeson and M. Riordan, March 15, 1992. BACK
39. SWW. BACK
40. SWW. BACK
41. SWW. BACK
42. SWW.BACK
43. SWW. BACK
44. D. Lazarus interview with I. Elichirigoity, 24 February 1992. BACK
45. J. Bardeen to S. A. Goudsmit, Feb. 15, 1957, Bardeen Papers, UIUC. BACK
46. J. Bardeen, L. N. Coooper, and J. R. Schrieffer, "Microscopic Theory of Superconductivity," Physical Review 106 (1957), 162-164. BACK
47. Charles Slichter, John Bardeen Memorial talk, February 8, 1991. BACK
48. Among the first were R. W. Morse and H. V. Bohm at Brown and Glover and Tinkham at Berkeley BACK
49. J. Bardeen, L.N. Cooper, and J. R. Schrieffer, "Theory of Superconductivity," Physical Review, Vol. 108 (1957), 1175-1204. BACK
50. L. Brown, R. Brout, T. Y. Cao, Peter Higgs, and Y. Nambu, "Panel Session: Spontaneous Breaking of Symmetry, L. Hoddeson, L. M. Brown, M. Dresden, and M. Riordan (eds), The Rise of the Standard Model: Particle Physics in the 1960s and 70s (New York: Cambridge Univ. Press, 1997), pp. 478-522.
